Q1. In a pure semiconductor crystal of Si, if antimony is added then what type of extrinsic semiconductor
is obtained. Draw the energy band diagram of this extrinsic semiconductor so formed.
ANS 1
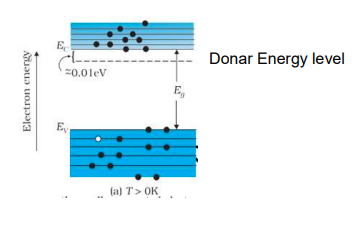
As given in the statement antimony is added to pure Si crystal,
then a n -type extrinsic semiconductor would be so obtained,
Since antimony(Sb) is a pentavalent impurity. Energy level diagram of n-type semiconductor
Q2. Consider two different hydrogen atoms. The electron in each atom is in an excited state. Is it possible for the electrons to have different energies but same orbital angular momentum according to the Bohr model? Justify your answer.
OR
Explain how does (i) photoelectric current and (ii) kinetic energy of the photoelectrons emitted in a photocell vary if the frequency of incident radiation is doubled, but keeping the intensity same? Show the graphical variation in the above two cases.
ANS 2 No
Because according to Bohr’s model,
En = − ଵଷ. ୬మ
and electrons having different energies belong to different levels having different values of n. #So, their angular momenta will be different, as
L = mvr = ୬୦ ଶ
OR
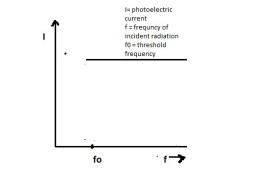
(i) The increase in the frequency of incident radiation has no effect on
photoelectric current. This is because of incident photon of 1/2 increased energy cannot eject more than one electron from the
metal surface.
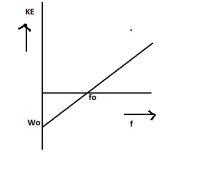
(ii) The kinetic energy of the photoelectron becomes more than the
double of its original energy. As the work function of the metal is fixed, so incident photon of higher frequency and hence higher energy will impart more energy to the photoelectrons.
Q3. Name the device which converts the change in intensity of illumination to change in electric current
flowing through it. Plot I-V characteristics of this device for different intensities. State any two applications of this device.
ANS 3 Photodiodes are used to detect optical signals of different
intensities by changing current flowing through them.
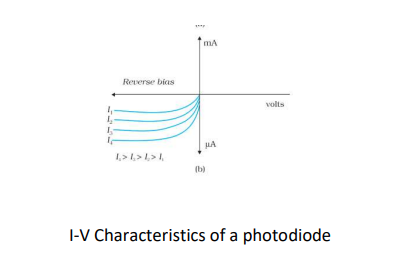
Applications of photodiodes:
1. In detection of optical signals.
2. In demodulation of optical signals.
3. In light operated switches.
4. In speed reading of computer punched cards.
5. In electronic counters
Q4. Derive an expression for the frequency of radiation emitted when a hydrogen atom de-excites from level n to level (n – 1). Also show that for large values of n, this frequency equals to classical frequency of revolution of an electron.
ANS 4 From Bohr’s theory, the frequency f of the radiation emitted when an electron de – excites from level nଶ to level nଵis given as
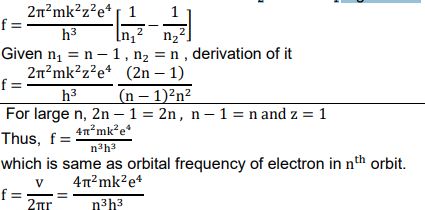
Q5. Explain with a proper diagram how an ac signal can be converted into dc ( pulsating)signal with output frequency as double than the input frequency using pn junction diode. Give its input and output waveforms.
ANS 5 A junction diode allows current to pass only when it is forward
biased. So, if an alternating voltage is applied across a diode the
current flows only in that part of the cycle when the diode is forward biased. This property is used to rectify alternating voltages and the circuit used for this purpose is called a rectifier.
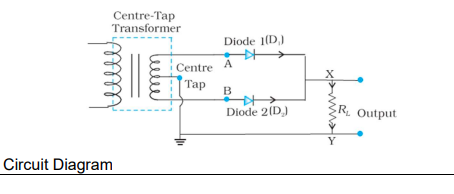
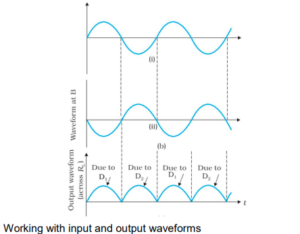
Q6. How long can an electric lamp of 100 W be kept glowing by fusion of 2 kg of deuterium? Take the fusion reaction as
![]()
ANS 6 Number of atoms present in 2 g of deuterium = 6 × 10ଶଷ
Number of atoms present in 2.0 Kg of deuterium = 6 × 10ଶ
Energy released in fusion of 2 deuterium atoms
= 3.27 MeV Energy released in fusion of 2.0 Kg of deuterium atoms
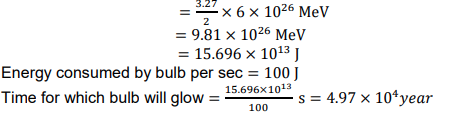
Q7. Define wavefront. Draw the shape of refracted wavefront when the plane incident wave undergoes
refraction from optically denser medium to rarer medium. Hence prove Snell’s law of refraction.
ANS 7 A locus of points, which oscillate in phase is called a wavefront.
OR
A wavefront is defined as a surface of constant phase.
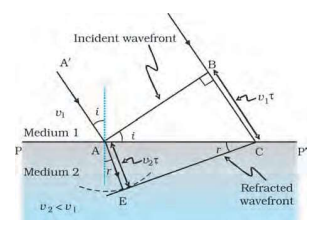
Diagram
Proof nଵ sin i = nଶ sin r (Derivation)
This is the Snell’s law of refraction.
Q8. (a) Draw a ray diagram of compound microscope for the final image formed at least distance of distinct vision?
(b) An angular magnification of 30X is desired using an objective of focal length 1.25 cm and an eye piece of focal length 5 cm. How will you set up the compound microscope for the final image formed at least distance of distinct vision?
OR
(a) Draw a ray diagram of Astronomical Telescope for the final image formed at infinity.
(b) A small telescope has an objective lens of focal length 140 cm and an eyepiece of focal length 5.0 cm. Find the magnifying power of the telescope for viewing distant objects when
(i) the telescope is in normal adjustment,
(ii) the final image is formed at the least distance of distinct vision.
ANS 8
(a)
Diagram of Compound Microscope for the final image formed at D:
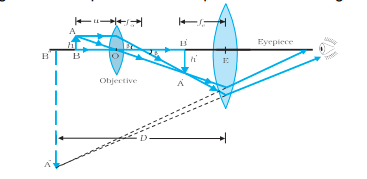
(b) m୭ = 30, f୭ = 1.25 cm, fୣ = 5 cm
when image is formed at least distance of distinct vision, D = 25cm
Angular magnification of eyepiece
![]()

As the objective lens forms the real image,
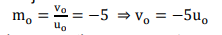
using lens equation, u୭ = −1.5 cm, v୭ = −5 × (−1.5)cm = +7.5 cm
Given vୣ = −D = −25 cm, fୣ = +5 cm, uୣ = ? using again lens equation uୣ =
ଶହ
Thus, object is to be placed at 1.5 cm from the objective and separation between the two lenses should be
L = v୭ + Iuୣ
l = 11.67 cm
OR
ANS 8
(a) Ray diagram of astronomical telescope when image is formed at
infinity.
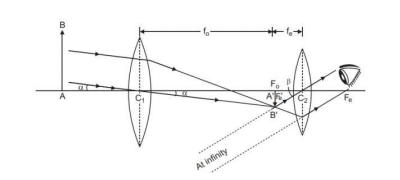
(b) (i) In normal adjustment : Magnifying power.
m = f୭/fୣ = (140/5) = 28
(ii) When the final image is formed at the least distance of distinct vision (25 cm) :
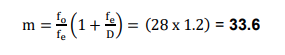
Q9. Light of wavelength 2000 Å falls on a metal surface of work function 4.2 eV.
(a) What is the kinetic energy (in eV) of the fastest electrons emitted from the surface?
(b) What will be the change in the energy of the emitted electrons if the intensity of light with same wavelength is doubled?
(c) If the same light falls on another surface of work function 6.5 eV, what will be the energy of emitted electrons?
ANS 9
λ = 2000 Å = (2000 × 10ିଵ)m
W୭ = 4.2eV
h = 6.63 × 10ିଷସJS
(a) Using Einstein’s photoelectric equation
K. E. = (6.2 – 4.2) eV = 2.0 eV
(b) The energy of the emitted electrons does not depend upon
intensity of incident light; hence the energy remains unchanged.
(c) For this surface, electrons will not be emitted as the energy of
incident light (6.2 eV) is less than the work function (6.5 eV) of the
surface.
Q10. The focal length of a convex lens made of glass of refractive index (1.5) is 20 cm. What will be its new focal length when placed in a medium of refractive index 1.25 ?
Is focal length positive or negative? What does it signify?
ANS 10 Given aஜౝ = 1.5
Focal length of the given convex lens when it is placed in air is
f = + 20 cm Refractive index of the given medium with respect to air is
aஜౣ = 1.25 New focal length of the given convex lens when placed in a
medium is f
ᇱ
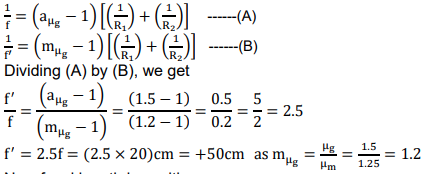
New focal length is positive. The significance of the positive sign of the focal length is that given convex lens is still converging in the given medium.
Q11. (a) Name the e.m. waves which are suitable for radar systems used in aircraft navigation. Write the range of frequency of these waves.
(b) If the Earth did not have atmosphere, would its average surface temperature be higher or lower than what it is now? Explain.
(c) An e.m. wave exerts pressure on the surface on which it is incident. Justify.
OR
(a) “If the slits in Young’s double slit experiment are identical, then intensity at any point on the screen may vary between zero and four times to the intensity due to single slit”. Justify the above statement through a relevant mathematical expression.
(b) Draw the intensity distribution as function of phase angle when diffraction of light takes place through coherently illuminated single slit.
ANS 11.
(a) Microwaves are suitable for the radar system used in aircraft
navigation. Range of frequency of microwaves is 108 Hz to 1011 Hz.
(b) If the Earth did not have atmosphere, then there would be absence
of greenhouse effect of the atmosphere. Due to this reason, the temperature of the earth would be lower than what it is now.
(c) An e.m. wave carries momentum with itself and given by
P = Energy of wave(U)/ Speed of the wave(c)
= U/c when it is incident upon a surface it exerts pressure on it.
OR
ANS. 11
(a)
The total intensity at a point where the phase difference is ∅, is
given by 𝐼 = 𝐼ଵ + 𝐼ଶ + 2ඥ𝐼ଵ𝐼ଶ 𝐶𝑂𝑆 ∅. Here 𝐼ଵ and 𝐼ଶ are the intensities of two individual sources which are equal.
When ∅ is 0, I = 4𝐼ଵ.
When ∅ is 90o
, I = 0
Thus intensity on the screen varies between 4𝐼ଵ and 0.
ANS. 11
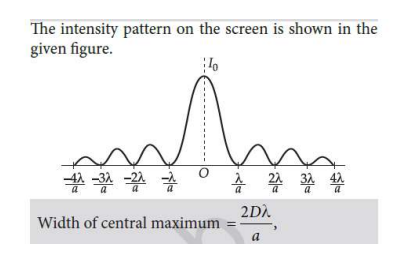
(b)
Intensity distribution as function of phase angle, when diffraction of
light takes place through coherently illuminated single slit
Q12. CASE STUDY: MIRAGE IN DESERTS
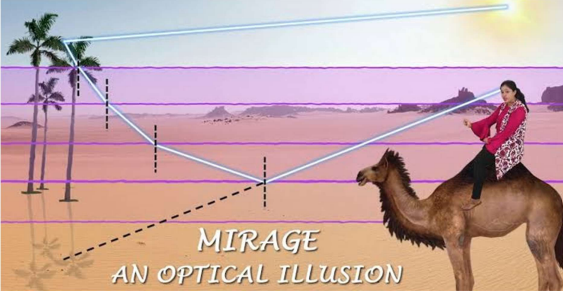

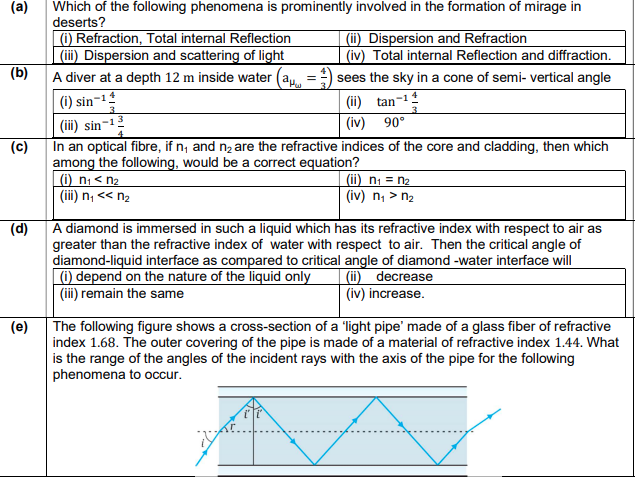

ANS 12.
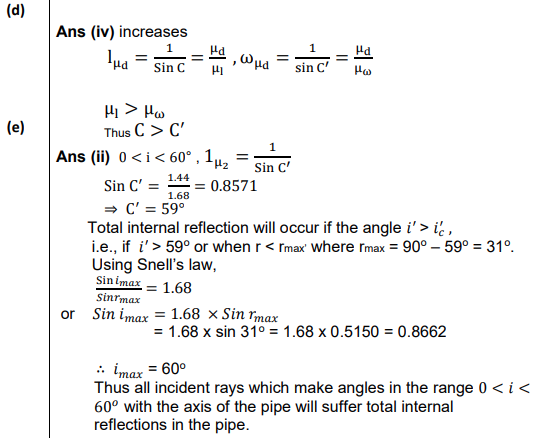
(a) Ans (i) Refraction, Total internal reflection
(b)
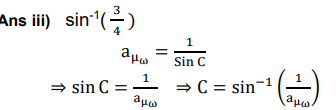
(c) Ans (iv) n1 > n2
The refractive index of the core should be greater than the refractive index of the cladding.